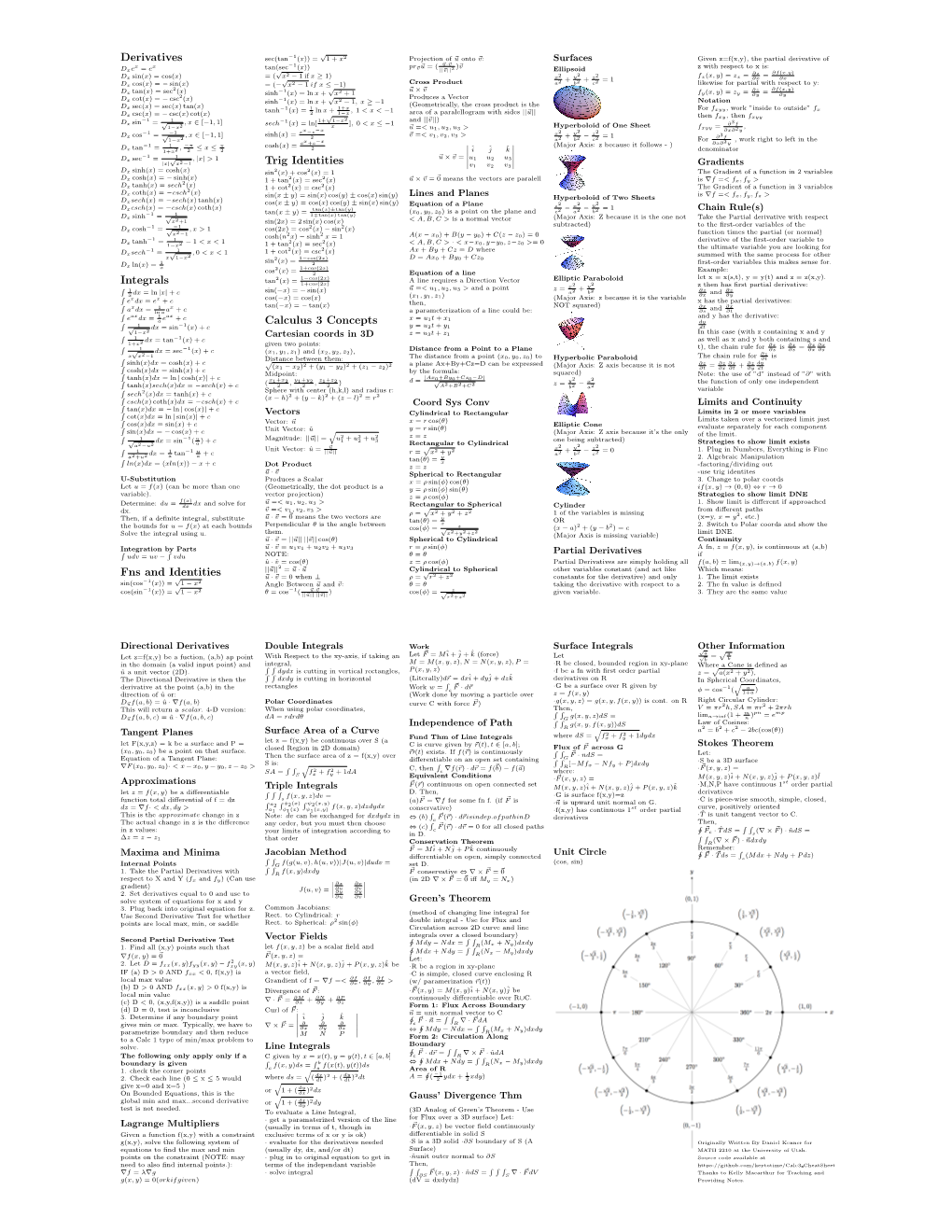
Multivariable Calc Cheat Sheet
Multivariable Calc Cheat Sheet - If point is de ned, limit exists. Compare this result to approaching. Check each line (0 x 5would give x=0. Find and classify the critical points of the following functions: The following only apply only if a boundary is given 1. (a) f(x,y) = x5+y4−5x−32y+81 (b) f(x,y) = x3+y3+3xy−27 (c) f(x,y) = x2y+3xy−3x2−4x+2y+1 2. Check the corner points 2. Substitute in for x and y. Web to a calc 1 type of min/max problem to solve.
Check the corner points 2. Find and classify the critical points of the following functions: Web to a calc 1 type of min/max problem to solve. Substitute in for x and y. If point is de ned, limit exists. (a) f(x,y) = x5+y4−5x−32y+81 (b) f(x,y) = x3+y3+3xy−27 (c) f(x,y) = x2y+3xy−3x2−4x+2y+1 2. Check each line (0 x 5would give x=0. The following only apply only if a boundary is given 1. Compare this result to approaching.
Web to a calc 1 type of min/max problem to solve. Check each line (0 x 5would give x=0. If point is de ned, limit exists. The following only apply only if a boundary is given 1. Check the corner points 2. Find and classify the critical points of the following functions: Substitute in for x and y. (a) f(x,y) = x5+y4−5x−32y+81 (b) f(x,y) = x3+y3+3xy−27 (c) f(x,y) = x2y+3xy−3x2−4x+2y+1 2. Compare this result to approaching.
Multivariable Calculus Cheat Sheet Reduced DocsLib
Substitute in for x and y. Check the corner points 2. Find and classify the critical points of the following functions: If point is de ned, limit exists. Check each line (0 x 5would give x=0.
Multivariable calculus cheat sheet pdf United States Manuals Working
Check each line (0 x 5would give x=0. Check the corner points 2. If point is de ned, limit exists. Find and classify the critical points of the following functions: Substitute in for x and y.
Hiset Math Cheat Sheet A Quick Review And A Complete List Of Ged
Web to a calc 1 type of min/max problem to solve. If point is de ned, limit exists. Find and classify the critical points of the following functions: The following only apply only if a boundary is given 1. Check the corner points 2.
Chain Rule in Multivariable Calculus made easy Mathematics Stack Exchange
(a) f(x,y) = x5+y4−5x−32y+81 (b) f(x,y) = x3+y3+3xy−27 (c) f(x,y) = x2y+3xy−3x2−4x+2y+1 2. Check each line (0 x 5would give x=0. If point is de ned, limit exists. The following only apply only if a boundary is given 1. Web to a calc 1 type of min/max problem to solve.
multivariable calculus how to classify x^24xy 2xz+z^2=1
The following only apply only if a boundary is given 1. If point is de ned, limit exists. Substitute in for x and y. Check the corner points 2. Find and classify the critical points of the following functions:
Vector Calc 4 Math cheat sheet, Physics and mathematics, Studying math
If point is de ned, limit exists. Find and classify the critical points of the following functions: (a) f(x,y) = x5+y4−5x−32y+81 (b) f(x,y) = x3+y3+3xy−27 (c) f(x,y) = x2y+3xy−3x2−4x+2y+1 2. Substitute in for x and y. Check the corner points 2.
calculus cheat sheet PreCal & Calculus Pinterest
Find and classify the critical points of the following functions: Compare this result to approaching. Check the corner points 2. Check each line (0 x 5would give x=0. If point is de ned, limit exists.
Calc 2 Sequences and Series Algebra Review, Math Review, Algebra 1
Web to a calc 1 type of min/max problem to solve. (a) f(x,y) = x5+y4−5x−32y+81 (b) f(x,y) = x3+y3+3xy−27 (c) f(x,y) = x2y+3xy−3x2−4x+2y+1 2. Check the corner points 2. Substitute in for x and y. Find and classify the critical points of the following functions:
Vector Calc 4 Calculus, Math cheat sheet, Math formulas
Web to a calc 1 type of min/max problem to solve. Substitute in for x and y. Check the corner points 2. The following only apply only if a boundary is given 1. Compare this result to approaching.
vector calculus Calculus, Vector calculus, Calculus notes
(a) f(x,y) = x5+y4−5x−32y+81 (b) f(x,y) = x3+y3+3xy−27 (c) f(x,y) = x2y+3xy−3x2−4x+2y+1 2. Find and classify the critical points of the following functions: Substitute in for x and y. Check the corner points 2. Web to a calc 1 type of min/max problem to solve.
The Following Only Apply Only If A Boundary Is Given 1.
Web to a calc 1 type of min/max problem to solve. Substitute in for x and y. Check each line (0 x 5would give x=0. If point is de ned, limit exists.
Check The Corner Points 2.
Find and classify the critical points of the following functions: Compare this result to approaching. (a) f(x,y) = x5+y4−5x−32y+81 (b) f(x,y) = x3+y3+3xy−27 (c) f(x,y) = x2y+3xy−3x2−4x+2y+1 2.